
EMAT 6600
Problem Solving
How wide is the
Alley?
Problem: Two buildings are separated by an alley. Two ladders are placed so that the base of each ladder is against one of the buildings and reaches the top of the other building. The two ladders are 40 feet and 30 feet long. Further, they cross at a point 10 feet from the ground. How wide is the alley?
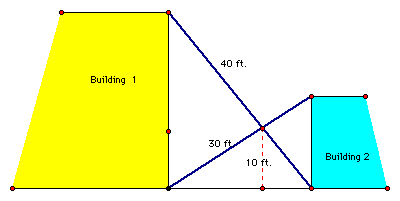
Solution:
Consider the following,

we then have the equation 30/x = 40/y which implies that y = 4x/3.
Using the Pythagorean theorem, 1600 – (16x2/9) is the length of the alley squared.
Therefore,
1600 – (16x2/9) + x2 = 900
- x2 (25/9) = - 700
- 25x2 = - 6300
x2 = 6300/25
x2 = 252
x = 2Ö73.
By substitution, 900 – 252 = 648.
The length of the valley is Ö648 = 18Ö2.
I would say that yes, there is a consistent construction because the lengths of the diagonals are given and we know that they cross at 10 feet above the ground at all times. With the given, the construction does not alter very much except the length of the buildings. Click here to see construction.